Extremal graphs and a conjecture of Erdos-Simonovits
Jie Ma
(University of Science and Technology of China)
Abstract: In extremal graph theory one is interested in the realtions between various graph invariants. Given a property P and an invariant u for a family F of graphs, we wish to determine the maximum value of u(G) among all graphs G in F satisfying the property P. The optimial value u(G) is called the extremal number and the graphs attaining this value are called extremal graphs. A principal example of such an extremal problem is the so-called Turan type problem, initiated by Hungarian mathematicians Turan and Erdos in 1940s. In this talk, we will discuss some recent results on extremal graphs and a related conjecture of Erdos-Simonovits.
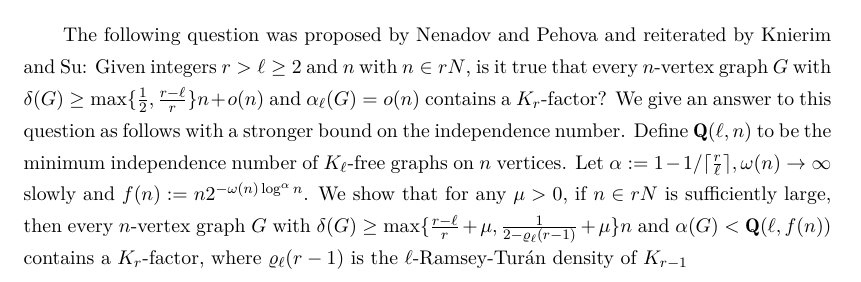
Clique factors of graphs with low independence number
Ping Hu
(Sun Yat-sen University)
A better bound on the size of rainbow matchings
Hongliang Lu
(Xi'an Jiaotong University)
